Overview
Our lab is interested in how cells solve geometry problems.
Cells have complex and beautiful structures that are required for function, and that become abnormal in disease. The goal of the lab is to determine how molecular processes determine geometry at the level of organelles and ultimately of whole cells. This is an interesting challenge due to the difference in size scale between molecular interactions and cellular structures.
Two of our primary approaches for understanding the origins of cell geometry are: (A) analyzing the mechanisms of organelle size control, using Chlamydomonas flagellar length control as a model system, and (B) exploring the mechanism of pattern formation and regeneration in single cells, using the giant cell Stentor coeruleus. We are also interested in questions about organelle arrangement and shape, both at the level of whole-cell anatomy and at the level of mitochondrial network morphology, both of which we are investigating in budding yeast. Our work on organelle size and shape raises the question of how these features could be re-engineered to change cellular function. Finally, we have been inspired by the complex structure of cells to explore the complexity of cell behavior and computational processes, viewing the cell as a robot that could ultimately be reprogrammed.
Our work uses an integrated combination of microscopy, genetics, genomics, molecular biology, and computational methods. Many of our experiments are driven by mathematical models, and we adopt new model organisms as necessary to answer specific questions.
Lab Philosophy - focus on questions
Because our lab is driven by conceptual questions and not by interest in any one specific organelle or model organism, we tend to take a variety of approaches, always seeking the simplest possible system in which to study a particular type of geometry problem. For example, in our study of organelle size, we began by studying length control of cilia and flagella, because these organelles are essentially one-dimensional, making size control particularly easy to study. We also do not hesitate to switch between model organisms as the need dictates. For studying cilia and flagella, we mostly use Chlamydomonas reinhardtii, a green algae with genetics similar to yeast. We choose this organism because it is the easiest organism to work with that actually has cilia and flagella (yeast, regrettably, do not). However, we also look at cilia in mammalian cells which, while harder to work with than Chlamydomonas, have other advantages in terms of the molecular tools and knowledge about disease-related mutations. For questions about global cell patterning, we turn to Stentor coeruleus, a giant ciliate with conspicuous surface patterning and a remarkable ability to heal wounds, which allows us to study how cells regenerate. Another approach we are taking to global cell geometry is to explore the role of physical packing interactions between organelles in yeast, to ask how simple steric packing may influence organelle size, shape, and membrane contacts. As other specific questions arise, we are free to adopt other specific organisms as necessary to let us answer the question at hand as easily and directly as possible. Examples include using Euplotes to study computational processes coordinating cell movement, or budding yeast to study the morphology of mitochondrial networks.
Current Projects in the Lab
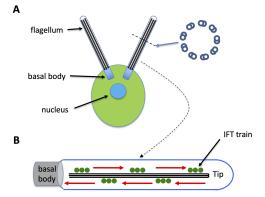
How Cells Control Organelle Size
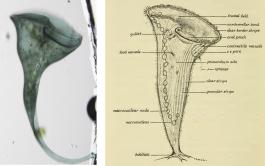
Pattern Formation and Regeneration in a Single Cell
How packing of organelles affects their shape and membrane contacts
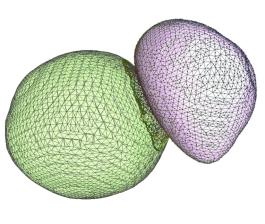
This image shows the vacuole and nucleus of a budding yeast cell. Because there is only limited volume inside the cell, there is no way to pack both organelles into the cell and have them remain spherical. As a result, the nucleus becomes deformed. This same packing constraint also means that the two organelles will always be in physical contact, since there isnt enough room to have them both fit into the cell interior without them touching. We are interested in how these packing constraints influence organelle shape and position, and also the role they may play in driving organelle-organelle membrane contacts.
Geometry of organelle shapes
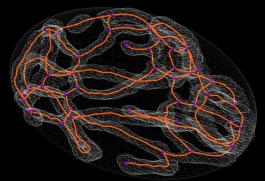
While some organelles are approximately spherical, others can have very complex shapes. Mitochondria resemble mathematical graphs, and this raises the question of what class of graphs represent mitochondrial networks. A second graph theory question is how the various morphological processes of mitochondria like fission and fusion sculpt the set of networks seen in cells.